1. Introduction

The Lorenz attractor, a mathematical model derived from the study of atmospheric convection, has intrigued scientists since its discovery because of its ability to demonstrate chaotic behavior in deterministic systems. Over the years, significant progress has been made in starting points based on its fundamental properties, such as sensitivity to initial conditions and the emergence of complex patterns in phase space (a famous formulation of chaos theory). In 2021, Syukuro Manabe and Klaus Hasselmann were awarded the Nobel Prize in Physics [1]. Therefore, we should pay more attention to the application of Lorenz attractors, especially the potential of practical implementation.

This paper aims to fill in these gaps by conducting a comprehensive exploration of the properties of the Lorenz attractor and delving into its various fields of application. Specifically, it attempts to illustrate the utility in simulating real-world phenomena, as well as its potential for new applications in areas such as data encryption and signal processing, by explaining problems related to the behavior of the attractor under different parameter regimes.

To achieve these goals, this study employs a multifaceted approach that combines mathematical analysis with numerical simulations. This approach not only allows to gain a deeper understanding of the attractor dynamics, but also to test its applicability in real-world environments.

The significance of this research lies in its potential to advance our understanding of chaotic systems. By exploring the properties and applications of Lorenz attractors, this paper paves the way for future research in areas such as climate modeling, where chaotic dynamics play a crucial role. In addition, the findings provide valuable insights for engineers and scientists seeking to exploit chaos for innovative technologies, such as secure communication systems. Ultimately, this work contributes to the development of the field of chaos theory, providing a reference point for future research, and providing avenues for further exploration [2].

2. The basic concepts and properties of Lorenz attractors

Lorenz attractors are mathematical models that describe the behavior of nonlinear dynamical systems in three-dimensional space. It gets its name from meteorologist and mathematician Edward Lorenz, who simplified the fluid dynamics equations while studying atmospheric convection, resulting in this mathematical model describing chaotic behavior [3].

The basic concept of Lorenz attractors consists of three key elements: aperiodicity in deterministic systems, sensitive dependence on initial conditions, and fractal structure [1].

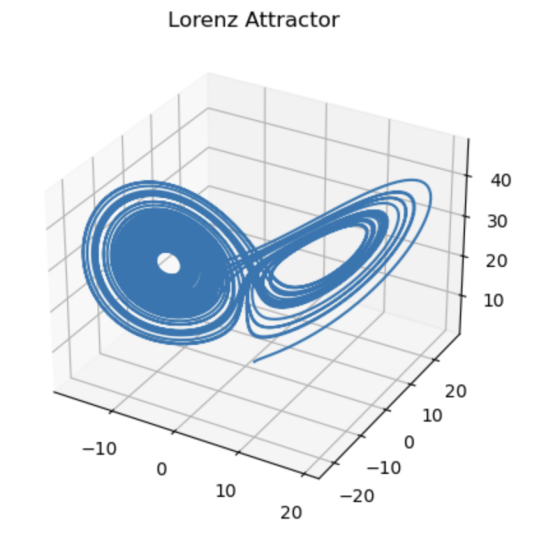
Figure 1. Lorenz Attactor

Non-periodicity in deterministic systems, i.e., unpredictability: A Lorenz attractor is a deterministic system in which it is generally assumed that the future state of the system can be fully determined given initial conditions (Figure 1). Lorenz attractors, however, show that even in a fully deterministic system, unpredictable long-term behavior can occur.

The aperiodicity of a Lorenz attractor is reflected in the trajectory of its state points in three-dimensional phase space. Instead of forming simple periodic patterns, these trajectories exhibit complex and seemingly random structures. Although the system is governed by deterministic equations, the motion of the state points is disordered and unpredictable.

The reason for this aperiodic behavior is that the sensitivity of the system depends on the initial conditions. In Lorenz attractors, even small changes in initial conditions can lead to large differences in the state of the system over time. This “butterfly effect” makes long-term predictions impossible, as any small error is amplified by the system, ultimately leading to a completely different outcome.

The non-periodicity of Lorenz attractors reveals the essential feature of chaotic systems, that is, the inherent randomness in deterministic systems. This randomness does not come from external noise or uncertainty factors, but is generated by the nonlinear dynamic mechanism inside the system. This led us to revisit the predictability of deterministic systems and to recognize that in some cases, even with perfect models and precise initial conditions, the long-term behavior of the system cannot be accurately predicted.

Therefore, aperiodicity is an important feature of chaos theory, and the Lorenz attractor is a famous representative of chaos theory, which challenges our traditional understanding of deterministic systems and provides new perspectives and methods for studying the behavior of complex systems [4].

2.1. Sensitive dependence on initial conditions

This dependence is reflected in the fact that even very small changes to initial conditions can lead to huge differences in the evolution of the system over time. This property makes the Lorenz attractor a typical representative of chaos theory and reveals the possible inherent randomness and impossibility of long-term prediction in deterministic systems.

Specifically, Lorenz attractors are described by a set of nonlinear differential equations that determine the dynamic behavior of a system in phase space. When there are small changes in the initial conditions, these small differences are gradually amplified due to the nonlinear nature of the system, eventually resulting in a completely different trajectory of the system state in phase space. This separation of trajectories is exponential, meaning that the differences grow rapidly over time.

This sensitive dependence on initial conditions is of great significance in practical applications. In climate forecasting, for example, small changes in initial conditions can lead to completely different weather patterns, making accurate long-term predictions difficult. Similarly, in other complex systems, such as economic, ecological, and social systems, small changes in initial conditions can trigger large chain reactions and unpredictable results.

This property of the Lorenz attractor also suggests that we must be careful about the initial conditions and the accuracy of the data when studying and dealing with complex systems. Even small errors or uncertainties can lead us to misunderstand and mispredict the behavior of the system. Therefore, the sensitive dependence on initial conditions is an important factor in chaos theory and complex system research [5].

2.2. Fractal structure

The fractal structure of the Lorenz attractor is a manifestation of its chaotic nature. Fractal is a class of geometric objects with fine structure, self-similarity and fractional dimension. It has a complex fractal structure in phase space. A fractal is a mathematical object with self-similarity and fine structure, and its dimension is usually not an integer. The trajectories of Lorenz attractors form a butterfly-shaped structure in three dimensions with infinite levels of self-similarity, i.e. similar patterns are seen at different scales. In the Lorenz attractor, its trajectories form a complex, butterfly-shaped structure in three-dimensional phase space that has fractal characteristics [6].

Specifically, the fractal structure of a Lorenz attractor is manifested in an infinite fold and nesting of its trajectories. Due to the chaotic nature of the system, the trajectory is constantly twisted and folded in the phase space, forming a self-similar structure. This self-similarity means that at different scales, people can all observe similar patterns and structures.

In addition, the fractal structure of the Lorenz attractor is also reflected in its dimensions. Although the trajectory is developed in three-dimensional space, due to its complexity and chaotic nature, its effective dimension is often an integer value, which is also an important feature of fractal structure.

The fractal structure of Lorenz attractors reveals the complexity and fine structure of chaotic systems, which provides an important perspective and tool for us to understand and study chaotic phenomena. At the same time, this fractal structure has also found a wide range of applications and inspiration in other fields such as art and computer science.

In general, the three properties of Lorenz attractors, aperiodicity in deterministic systems, sensitive dependence on initial conditions, and fractal structure are all interrelated, or are typical features of chaotic models.

3. The formation mechanism and mathematical principle of Lorenz attractor

The Lorenz attractor formation mechanism is mainly based on three key variables: \( x \) , \( y \) and \( z \) , which constitute the core dynamic elements in the convection process. Specifically, \( x \) stands for convective intensity, which reflects the accumulation and release of energy in the atmosphere; y represents the temperature difference between upwelling and downwelling, revealing the distribution and transport of heat in the atmosphere. \( z \) represents the change in the vertical temperature profile, reflecting the stability of the atmospheric junction and the vertical transport of energy. These three variables evolve through a set of coupled nonlinear differential equations, which together shape the complex structure of the Lorenz attractor.

\( \frac{dx}{dy}=σ(y-x) \ \ \ (1) \)

\( \frac{dy}{dt}=x(ρ-z)-y \ \ \ (2) \)

\( \frac{dz}{dt}=xy-βz \ \ \ (3) \)

Where σ is the Prandtl number, representing the ratio of the viscous effect to the heat conduction effect in fluid motion; ρ is the Rayleigh number, which reflects the balance between energy input and dissipation in the system. β is a temperature structure-related parameter that measures the difference in decay rates between horizontal and vertical temperature structures. These system parameters determine the dynamic behavior of the system. This set of equations describes the process of energy conversion and transport in atmospheric convection, which includes nonlinear interaction and feedback mechanisms [1].

When given appropriate parameter values, this set of equations will exhibit chaotic behavior. As mentioned above, chaos refers to the unpredictability of the state of the system over long time scales, and even small differences in initial conditions can lead to large changes in the state of the system. The numerical results are plotted in a three-dimensional phase space supported by \( x \) , \( y \) and \( z \) . This is a continuous smooth curve that seems to turn left and right disordered in three-dimensional space, it does not intersect itself, showing a complex structural pattern. No matter where the initial value is chosen, the system orbitals have the same destination, forming what is called a singular attractor. On the singular attractor, if two points that are arbitrarily close to each other are selected as the initial values, their motion paths are rapidly separated in an exponential manner, showing extreme sensitivity to the initial values.

The formation mechanism and mathematical principle of Lorenz attractor reveal the essential characteristics of chaotic phenomena, that is, the inherent randomness in deterministic systems and the impossibility of long-term prediction. This model not only has a wide range of applications in the field of atmospheric science, but also provides important theoretical tools and research ideas for other disciplines such as physics, mathematics and engineering. Through the in-depth study of Lorenz attractors, people can better understand and explore the dynamic behavior and evolution of complex systems in nature.

4. The application value of Lorenz attractor in chaos theory

Lorenz attractors have very important application value in chaos theory. First, as a classic example of chaos theory, Lorenz attractors deeply reveal the fundamental properties of chaotic systems, such as deterministic chaos, sensitive dependence on initial conditions, and fractal structure [7]. These properties are represented in many complex systems in the natural sciences and engineering, so by studying Lorenz attractors, people can better understand and describe the behavior of these systems.

Secondly, the mathematical model and image representation of Lorenz attractors provide a powerful tool for the study of chaos theory. Through the numerical simulation and visual analysis of Lorenz attractors, scientists can intuitively observe the formation process and dynamic evolution of chaotic phenomena, so as to further reveal the internal laws and operating mechanisms of chaotic systems.

In addition, Lorenz attractors have a wide range of value in practical applications. For example, in meteorology, the study of Lorenz attractors helps us understand complex changes in weather and climate, improving the accuracy of weather forecasts [8]. In terms of signal shielding, chaotic signals, due to their unpredictability, can be used to generate noise or interference signals to cover up information that needs to be kept secret. By adjusting the parameters of chaotic system, chaotic signals similar to but not identical to the original signal can be generated, so that the original signal can be shielded or hidden. This method has certain application value in protecting sensitive information from illegal interception or eavesdropping. In the aspect of secure communication, chaos theory provides a new idea for encryption technology.

Based on the sensitivity of a chaotic systems to initial conditions, encryption algorithms with high security can be designed. Specifically, the information to be encrypted can be used as the initial conditions or parameters of the chaotic system, and the ciphertext can be generated through the evolution of the chaotic system. Because the long-term behavior of chaotic systems is unpredictable, even if an attacker knows the encryption algorithm and part of the ciphertext information, it is difficult to infer the content of the original information. Therefore, the encryption algorithm based on chaos theory has a wide application prospect in the field of secure communication [9].

In conclusion, the application value of Lorenz attractors in chaos theory is reflected in many aspects, including in-depth understanding of chaos phenomena, providing research tools and promoting the development of practical applications. With the progress of science and technology, the application prospect of Lorenz attractor and chaos theory will be more and more broad.

5. Conclusion

Lorenz attractors have profoundly influenced our understanding of chaos theory and its applications in various fields of science. Through their complex properties, such as sensitivity to initial conditions, aperiodicity, and fractal structure, Lorenz attractors challenge traditional paradigms of predictability and determinism in dynamical systems.

This paper delves into the basic concepts and characteristics of the Lorenz attractor, emphasizes its role as a paradigm model in chaos theory. The sensitivity of attractors to small changes in initial conditions underscores the unpredictable nature of chaotic systems, a phenomenon often referred to as the “butterfly effect”. This property has important implications in fields such as meteorology, where small changes in initial data can lead to radically different weather patterns.

In addition, the fractal nature of Lorenz attractors, with their infinite complexity and self-similarity, provides insights into the intrinsic beauty and mathematical richness of chaotic systems. This fractal geometry has similarities in a variety of fields, from natural patterns in nature to designs in advanced technology.

In addition to its theoretical value, the Lorenz attractor is a practical tool in many applications. For example, in the field of encryption, the unpredictable trajectory of Lorenz systems is exploited to create robust and secure encryption algorithms. Similarly, in control systems engineering, an understanding of chaos theory helps to design more resilient and adaptable systems.

In summary, Lorenz’s attractors demonstrate a deep connection between mathematics, physics, and the natural world. Its properties and principles continue to inspire interdisciplinary researchers and push the boundaries of our understanding of chaos theory. As people further explore chaos theory, the Lorenz attractor remains a key reference point, guiding people through nonlinear dynamics into the fascinating field.