1. Introduction

Pascal’s Triangle, a set of numbers arranged in a triangular pattern - starting from 1 on the first row, 1 and 1 on the second row, with each row increasing by one number. It follows a certain property, that each number is the sum of the two numbers from the line above (if the numbers above are less than two, then this number is just 1). Pascal’s Pyramid, a three-dimensional extension of Pascal’s Triangle, which talks about the exact same properties. This paper uses the method of literature analysis and review, to explore the mathematical relationship between these two models and some of their applications, which can demonstrate their usefulness in the modern world.

2. Pascal’s Triangle

Pascal’s Triangle is essential for understanding binomial expansions, where each entry is calculated as the sum of the two numbers directly above it. This structure is important in combinatorial mathematics, as each row represents the coefficients of (x+y)^n, clarifying its importance in algebra and probability.

The triangle's elements are binomial coefficients, (nk) (n \choose k), which indicate the number of ways to select k elements from a set of n. With such, we can find the value of combination (n choose k) from the nth row, kth number of the Pascal’s Triangle. These coefficients in Pascal’s Triangle have broad applications, which can not only calculate probabilities in simple random events like coin tossing, but also prove algebraic identities such as the binomial theorem.

A huge amount of hidden properties can be found beyond this fundamental characteristic of Pascal’s Triangle. For example, the existence of the power of 2 appears in each row of Pascal’s Triangle. The first row is 1, which is 2^0; The second row is 1+1+2, which is 2^1, the third row is 1+2+1, which is 2^2, the fourth row is 1+3+3+1, which is 2^3, etc. It’s not easy to conclude the sum of nth row of Pascal’s Triangle is just 2^(n-1). Also, the power of 11 can be applied to Pascal’s Triangle. If we combine every number in each line into a brand-new number, it’s not easy to see the zeroth line, 1, which is 1*10^0=11^0; the first line, which is 1*10^1+1*10^0=11^1; the second line, which is 1*10^2+2*10^1+1*10^0=121, which is 11^2. By following this general rule, we can conclude for any line, denoted as p in Pascal’s Triangle, there’s a property that 1*10^p+(p choose 1)*10^(p-1)+(p choose 2)*10^(p-2)+…+1*10^0=11^p [1]. Moreover, Pascal’s Triangle has a strong connection with the Fibonacci sequence [2]. Details will be explained below.

This brief exploration of Pascal's Triangle highlights its multifaceted applications and its real-life application as well as its significance in mathematics-related problems, making it a subject of interest in both educational settings and advanced mathematical research.

3. Pascal’s Pyramid

Pascal’s Pyramid, also known as Pascal’s Tetrahedron, extends the principles of Pascal’s Triangle into three dimensions. Each layer of the pyramid can be seen as a separate Pascal’s Triangle, with one-row extension of the new layer of the pyramid, the numerical pattern of each layer in Pascal’s Pyramid is shown in Figure 1; however, it involves a more complex arrangement where each number is the sum of the three numbers directly above it in the previous layer. This arrangement not only demonstrates the geometric progression from two to three dimensions but also highlights the pyramid's foundational structure in combinatorial mathematics [3].

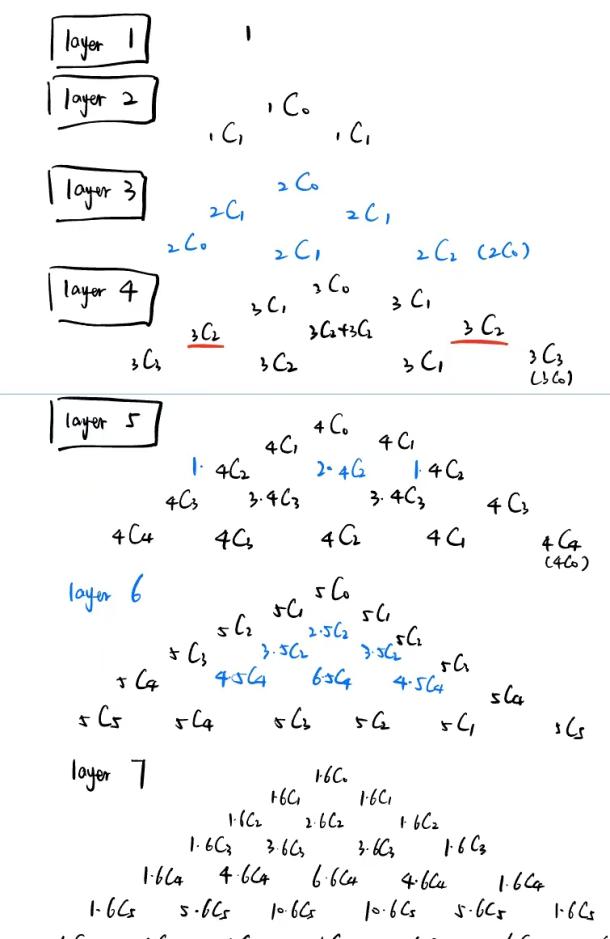
Figure 1: The numerical pattern of each layer in Pascal’s Pyramid

While Pascal’s Triangle can be used to express the coefficient of Binomial expansion, similarly, Pascal’s Pyramid can be used to express the coefficient of trinomial expansion. We already mentioned the connection between Pascal’s Triangle and the Fibonacci sequence, so it’s not easy to see that there’s also a connection between Pascal’s Pyramid and the Tribonacci sequence. Specifically, to define the Tribonacci sequence, we have T1=T2=1, and T3=2. By specify the value of T1, T2, and T3, we can find the value of Tn such that Tn=T(n-1)+T(n-2)+T(n-3). To apply this special property to Pascal’s Pyramid, we extend each Pascal’s Triangle on each layer of Pascal’s Pyramid line by line accordingly. As such, we form a new right triangle-shaped number set, with n lines starting with one number in the first row, and each row increasing by one number. By using this number set, we can find each Tribonacci number Dn in the sequence, which is represented by a diagonal line running from the bottom of the triangle up to the right. We can find any Tribonacci number Di by summing the corresponding diagonal in this triangle-shaped number set, starting from the ith line of the number set. For example, we can find the value of D4 (D4=D1+D2+D3=1+1+2=4) by summing the diagonal starting from the first number of the 4th line of the number set, so we get 1+1+2=4, which is exactly the value of D4 [4].

This concise overview of Pascal's Pyramid demonstrates how it extends Pascal's Triangle into three dimensions, with each layer representing an expanded triangle. By illustrating trinomial expansions - a more complex process compared to binomial coefficients, and their connection to the Tribonacci sequence, we can see its significance in mathematical contexts.

4. Generalization of Pascal’s Triangle: ab-based Model

While we can use Pascal’s Triangle to deal with the binomial expansion of (x+​y)^n, we can also extend the classical Pascal’s Triangle into a two-​parameter ab-​based model, which aims to find the binomial expansion of (ax+​by)^n.​ In this ab-​based triangle, how can we find the general formula of deriving each coefficient in each row? To start this, we take (3x+​4y)^4, which a=​3, b=​4 as an example.​ After computing this hand by hand, we find that (3x+​4y)^4=​81x^4+​432x^3y+​864x^2y^2+​768xy^3+​256y^4.​ By applying what we find from Pascal’s Triangle, we find that (3x+​4y)^4 can be written as (4 choose 0)(3x)^4(4y)^0+​(4 choose 1)(3x)^3(4y)^1+​(4 choose 2)(3x)^2(4y)^2+​(4 choose 3)(3x)^1(4y)^3+​(4 choose 4)(3x)^0(4y)^4 [5].​ Using what we find from this 34-​based Pascal’s triangle, we can get the general rule for the ab-​based triangle, which we used to expand (ax+​by)^n.​ The general formula is (n choose 0)(ax)^n(by)^0+​(n choose 1)(ax)^(n-​1)(by)^1+​(n choose 2)(ax)^(n-​2)(by)^2+​…+​(n choose n)(ax)^0(by)^n.​ We can write this in summation form, which is also called binomial expansion.​

5. Generalization of Pascal’s Pyramid: abc-based Model

Similarly, Pascal’s Pyramid can be also applied to form an abc-based model, which can be used to expand (ax+by+cz)^n. We also take an example of the abc-model to find its general formula. Suppose a=2, b=3, c=4, and n=4. After expanding this 234-based triangle, we get (2x+3y+4z)^4=16x^4+96x^3y+128x^3z+216x^2y^2+576x^2yz+384x^2z^2+216xy^3+864xyz^2+512xz^3+81y^4+432y^3z+864y^2z^2+768yz^3+256z^4. We can also rewrite this and get the general formula and write it in summation form, which is also called trinomial expansion [5].

In fact, such ab and abc-based models can be expanded infinitely, given the concept of multinomial expansion, which can not only just be used to expand complex equations, but also can be applied in probability theory, generating functions, as well as quantum mechanics and statistical analysis.

6. Conclusion

This paper explores the transition from Pascal's Triangle to Pascal's Pyramid, illustrating their significant role in understanding binomial, trinomial, and multinomial expansions by applying the ab and abc-based triangles. Additionally, this paper also indicates how people can connect Pascal's Triangle and Pascal's Pyramid to sequences like Fibonacci and Tribonacci, understanding the wide applicability of these concepts across various fields. While this paper has explored the binomial and trinomial expansions within Pascal's Triangle and Pascal's Pyramid respectively, it has not deeply delved into the complexities of multinomial expansions and the use of generating functions. Looking ahead, future research can further concentrate on a detailed exploration of generating functions, with its wide usage in mathematical applications, which could cover new applications and deeper insights into related properties.